Answer:
y =
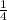 x + 9
x + 9
Explanation:
the equation of a line in slope- intercept form is
y = mx + c ( m is the slope and c the y- intercept )
given the line with equation
y = - 4x + 13 ← in slope- intercept form
with slope m = - 4
given a line with slope m then the slope of a line perpendicular to it is
 = -
= -
 = -
= -
 =
=
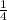
given this line has a y- intercept of 9, then c = 9
y =
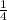 x + 9 ← equation of perpendicular line
x + 9 ← equation of perpendicular line