Question

rental car company find intersection of 2 equations and y-intercepts how would you graph that? they haul 40 and good deal 80?what number of miles driven does they haul charge more than good deal? slope equation for they haul?and slope equation for good deal?

Answer 1

Let x be the number of miles and y the total cost of the service.

Since the company They Haul charges $40 plus 0.20 per mile, then for x miles they would charge $40 plus 0.2x:

`y=0.2x+40`

Since the company Good Deal charges $80 regardless of the number of miles, then:

`y=80`

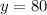
To find the intersection of both equations, set both expressions to be equal and solve for x:

`\begin{gathered} 0.2x+40=80 \\ \Rightarrow0.2x=80-40 \\ \Rightarrow0.2x=40 \\ \Rightarrow x=(40)/(0.2) \\ \Rightarrow x=200 \end{gathered}`

Then, the cost is the same for both companies if the amount of miles is equal to 200, and the cost would be $80.

To find the y-intercept for the company They Haul, evaluate the expression for x=0:

`\begin{gathered} y=0.2(0)+40 \\ \Rightarrow y=40 \end{gathered}`

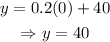
Since the expression for Good Deal does not depend on the value of x, then the y-intercept is:

`80`

To graph the equations:

`\begin{gathered} y=0.2x+40 \\ y=80 \end{gathered}`

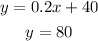
Find two points on each line and draw a line through those points.

To find a point on a line, substitute different values of x to find the corresponding values of y.

For instance, choose x=0 and x=100. From the first equation, we obtain the following values for y:

`\begin{gathered} x=0\Rightarrow y=0.2(0)+40 \\ \Rightarrow y=40 \\ x=10\Rightarrow y=0.2(100)+40 \\ \Rightarrow y=20+40=60 \end{gathered}`

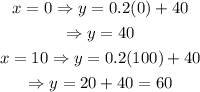
Then, the points (0,40) and (100,60) belong to the first line. Plot those two points on a coordinate plane and then draw a line through those points:

For the other line, the value of y is always 80 regardless of the value of x. Then, the points (50,80) and (150,80) belong to the line. Do the same procedure to draw that second line:

We can see that They Haul (red) charges more than Good Deal whenever the number of miles is greater than 200.

The slope-intercept form of the equation of a line with slope m and y-intercept b is:

`y=mx+b`

We already knew the equations for They Haul and Good Deal:

They Haul:

`y=0.2x+40`

The slope is equal to 0.2

Good Deal:

`y=80`

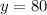
Since the x-variable does not appear, than means that the coefficient of x is 0, so the slope is equal to 0.