Question

Which of the following has a radius less than 5 inches? Circle the letter of all that apply.

(A) a cylindrical can with a volume of 424.12 cubic inches and a height of 15 inches

(B) a cylindrical can with a volume of 565.49 cubic inches and a height of 5 inches

(C) a cone with a volume of 201.06 cubic inches and a height of 12 inches

(D) a cone with a volume of 254.47 cubic inches and a height of 12 inches

Answer 1

The shapes with a radius less than 5 inches are (B) and (C).

Step-by-step explanation:

To find the radius of a cylinder or cone given their volumes and heights, you can use the formulas for their respective volumes:
`\(V_{\text{cylinder}} = \pi r^2 h\) and \(V_{\text{cone}} = (1)/(3) \pi r^2 h\), where \(r\) is the radius and \(h\) is the height. Rearranging these formulas, you get \(r = \sqrt{(V)/(\pi h)}\) for a cylinder and \(r = \sqrt[3]{(3V)/(\pi h)}\) for a cone.`

`For (A) with a volume of 424.12 cubic inches and a height of 15 inches, \(r = \sqrt{(424.12)/(\pi * 15)} \approx 4.37\) inches, so the radius is greater than 5 inches. For (B) with a volume of 565.49 cubic inches and a height of 5 inches, \(r = \sqrt{(565.49)/(\pi * 5)} \approx 4.76\) inches, which is less than 5 inches.`

`For (C) with a volume of 201.06 cubic inches and a height of 12 inches, \(r = \sqrt{(201.06)/(\pi * 12)} \approx 3.03\) inches, indicating a radius less than 5 inches. Finally, for (D) with a volume of 254.47 cubic inches and a height of 12 inches, \(r = \sqrt{(254.47)/(\pi * 12)} \approx 3.20\) inches, also showing a radius less than 5 inches.`

Answer 2

(B) and (D) have a radius less than 5 inches.

Step-by-step explanation:

To determine which of the given options has a radius less than 5 inches, we need to use the volume formulas for a cylinder and a cone. The formula for the volume of a cylinder is given by
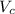`V_c` = π
`r_c^2`
`h_c`, where
`r_c` is the radius and
`h_c` is the height. Similarly, the formula for the volume of a cone is given by
`V_cone` = (1/3)π
`r_cone^2h_cone`, where
`r_cone` is the radius and
`h_cone` is the height.

For option (B), with a cylindrical can volume of 565.49 cubic inches and a height of 5 inches, we can rearrange the cylinder volume formula to solve for the radius:
`r_c`= √(
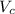`V_c`/ (π
`h_c`)). Plugging in the values, we find that
`r_c` ≈ √(565.49 / (π * 5)) ≈ 3 inches.

For option (D), with a cone volume of 254.47 cubic inches and a height of 12 inches, we can rearrange the cone volume formula to solve for the radius: r_cone = √((3 *
`V_cone`) / (π
`h_cone`)). Plugging in the values, we find that r_cone ≈ √((3 * 254.47) / (π * 12)) ≈ 4 inches.

Therefore, both (B) and (D) have a radius less than 5 inches, meeting the given criteria.