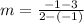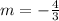Question

I'm confused apparently I have to use this graph to determine the instantaneous rate of change x= – 1 and I'm not exactly sure how to do that. I also have to determine the average rate of change on the interval -1 ≤ X ≤ 2

Answer 1

• The instantaneous rate of change at x= – 1 is 0 (zero).

• The average rate of change on the interval -1 ≤ x ≤ 2 is –4/3.

Step-by-step explanation

The instantaneous rate of change is the derivative at the point given. In our case, when x = –1, it is a maximum point and the tangent to this line is a constant y = –3 (tangent line represented by the blue line in the figure given). Thus, the derivative is 0, resulting in an instantaneous rate of change of 0:

Contrarily, the average rate of change on the interval –1 ≤ X ≤ 2 is the slope of the tangent line between points x = –1 and x = –2 (represented by the red line in the figure given), then, the slope can be calculated using the following formula:

`m=(y_2-y_1)/(x_2-x_1)`

Then, to use this formula we have to find the coordinates of these points:

Thus, the coordinates are (–1, 3) and (2, –1), by replacing these values we get:

`m=(-1-3)/(2-(-1))`
`m=(-4)/(2+1)`
`m=-(4)/(3)`