Question

The two lines below are parallel.y = 4 / 5x + 17y = 4 / 5x - 13true or false?

Answer 1

GIVEN:

We are given the following linear equations;

`\begin{gathered} y=(4)/(5)x+17 \\ \\ y=(4)/(5)x-13 \end{gathered}`

Required;

To determine if the two lines are parallel or not.

Step-by-step solution;

For any equation of a straight line given in the slope-intercept form, that is;

`y=mx+b`

Take note of the following variables;

`\begin{gathered} m=slope \\ \\ b=y-intercept \end{gathered}`

Observe carefully that the variable m is the coefficient of x. This simply means the coefficient of x is the slope (that is, m = slope).

Another important point to note here is that, where two lines have the same slope, then the two lines are parallel.

Therefore, we shall identify the slopes of both equations given as follows;

`\begin{gathered} For\text{ }y=(4)/(5)x+17 \\ \\ slope(m_1)=(4)/(5) \\ \\ For\text{ }y=(4)/(5)x-13 \\ \\ slope(m_2)=(4)/(5) \end{gathered}`

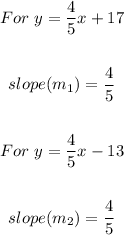
The value of the slope is the same for both equations which simply means the lines are parallel.

Therefore,

ANSWER:

TRUE