Question

A,E, and D are collinear. BCDE is a parallelogram. Find m

Answer 1

The answer would be be 29 degrees

Explanation:

Answer 2

B. 29º

Explanation:

Given that BCDE is a parallelogram, then
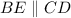`BE \parallel CD` and A, E and D are collinear. Then, angle E inside triangle ABE and angle D inside parallelogram BCDE have the same measure. That is:

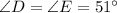`\angle D = \angle E = 51^(\circ)` (1)

In addition, the sum of internal angles in triangles equals 180º, which implies that:

`\angle A + \angle B + \angle E = 180^(\circ)` (2)

If we know that
`\angle B = 100^(\circ)` and
`\angle E = 51^(\circ)`, then
`\angle A` is:

`\angle A = 180^(\circ)-\angle B - \angle E`

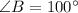`\angle A = 180^(\circ)-100^(\circ)-51^(\circ)`

`\angle A = 29^(\circ)`

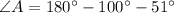
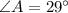
Hence, correct answer is B.