The equation that describes g(x) is g(x) = -2x
Why is this correct?
Upon analyzing the graph of the function
 , the following properties are evident:
, the following properties are evident:
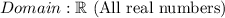
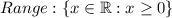
The reflection of
 over the x-axis yields a function that represents the inverse of
over the x-axis yields a function that represents the inverse of
 , denoted as
, denoted as
 .
.
The subsequent transformations are:
![\[ g(x) = -f(x) = [2x] = -2x \]](https://img.qammunity.org/2024/formulas/chemistry/high-school/jmytbmk9fy4i10djsdpuug7a8iw7db7mka.png) (utilizing absolute value properties)
(utilizing absolute value properties)
Hence, the transformed function
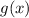 simplifies to
simplifies to
 after reflecting
after reflecting
 over the x-axis.
over the x-axis.
This transformation captures the manipulation applied to the original function
 , resulting in a reflected expression represented by
, resulting in a reflected expression represented by
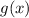 .
.