We have the expressions:
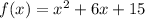
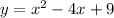

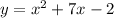
Now, with this we operate as follows:
a)

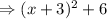
Then, the axis is x = -3 and the vertex (-3, 6)
b)
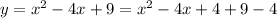
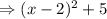
Then, the vertex is (2, 5) and the axis is x = 2.
c)
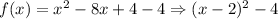
Then, the vertex is (2, -4) andd the axis is x = 2.
d)
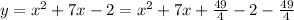
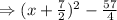
Then, the vertex is (-7/2, -57/4) and the axis is -7/2.