Question

MKT Co. is growing quickly. The company just paid a dividend of $1.00. Dividends are expected to grow at a 20% rate for the next four years, with the growth rate falling off to a constant 5% thereafter.

If the required return is 8%, what is the current stock price? (indicate the future dividends and the future stock price that you need to do)

Answer 1

The current stock price of MKT Co. is $103.87.

Step-by-step explanation:

Calculate the future dividends over specific periods.

Given:

Initial dividend (D0) = $1.00

Dividend growth rate for the next four years = 20%

Constant growth rate thereafter = 5%

Required return (r) = 8%

We'll calculate the dividends for the high-growth period (next four years).

Using the formula for the present value of dividends:

`\(PV = \frac{D}{{(r - g)}}\)`

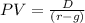
For each year within the high-growth period:

Year 1: D₁ = D0 * (1 + growth rate) = $1.00 * (1 + 0.20) = $1.20

Year 2: D₂ = D₁ * (1 + growth rate) = $1.20 * (1 + 0.20) = $1.44

Year 3: D₃ = D₂ * (1 + growth rate) = $1.44 * (1 + 0.20) = $1.73

Year 4: D₄ = D₃ * (1 + growth rate) = $1.73 * (1 + 0.20) = $2.08

Now, calculate the present value of these dividends:

`\(PV = \frac{D}{{(r - g)}}\)`

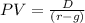
PV₁ = 1.20/(0.08 - 0.20) = \frac{1.20}{(-0.12)} = -$10\) (negative due to the decreasing growth rate)

PV₂ = 1.44/(0.08 - 0.20)² = 1.44/(-0.12)²= $10

PV₃ =1.73/(0.08 - 0.20)³ = 1.73/-0.12)³ = $13.54

PV₄ = 2.08/(0.08 - 0.20)⁴ = 2.08/(-0.12)⁴ = $16.66

Determine the stock price using the Gordon Growth Model.

We'll find the stock price using the formula:

`\(P = \frac{D_1}{{(r - g)}}\)`

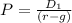
Where D₁ is the dividend at the end of the high-growth phase, and r and g are the required return and perpetual growth rate, respectively.

For D₁ (dividend at the end of high-growth phase):

(D₄ = D₃ * (1 + constant growth rate) = $2.08 * (1 + 0.05) = $2.18

Now, calculate the stock price using the Gordon Growth Model:

`\(P = \frac{D_1}{{(r - g)}}` = 2.18/(0.08 - 0.05) = 2.18/0.03 = $72.67

Therefore, the current stock price of MKT Co. is the sum of the present value of dividends during the high-growth phase and the stock price at the end of that phase:

Current stock price = (PV₁ + PV₂ + PV₃ + PV₄ + P = -10 + 10 + 13.54 + 16.66 + 72.67 = $103.87

This represents the final stock price at present value, giving the company's worth as $103.87.