Question

Given the graphs of g(x) & f(x),Find the following listed below

Answer 1

We are given the graphs of the functions g(x) and f(x)

a) (g+f)(1)

it can be written as

`(g+f)(1)=g(1)+f(1)`

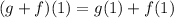
From the graph, we see that the values of the two functions at x = 1 are

g(1) = -1

f(1) = 1

`\begin{gathered} (g+f)(1)=g(1)+f(1) \\ (g+f)(1)=-1+1 \\ (g+f)(1)=0 \end{gathered}`

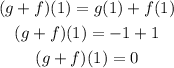
Therefore, (g+f)(1) = 0

b) (f-g)(-1)

It can be written as

`(f-g)(-1)=f(-1)-g(-1)`

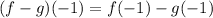
From the graph, we see that the values of the two functions at x = -1 are

g(-1) = 3

f(-1) = -1

`\begin{gathered} (f-g)(-1)=f(-1)-g(-1) \\ (f-g)(-1)=-1-3 \\ (f-g)(-1)=-4 \end{gathered}`

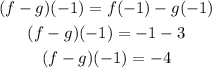
Therefore, (f-g)(-1) = -4

c) (g*f)(1)

It can be written as

`(g\cdot f)(1)=g(1)\cdot f(1)`

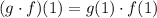
From the graph, we see that the values of the two functions at x = 1 are

g(1) = -1

f(1) = 1

`\begin{gathered} (g\cdot f)(1)=g(1)\cdot f(1) \\ (g\cdot f)(1)=-1\cdot1 \\ (g\cdot f)(1)=-1 \end{gathered}`

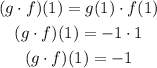
Therefore, (g*f)(1) = -1

d) (f/g)(4)

It can be written as

`((f)/(g))(4)=(f(4))/(g(4))`

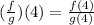
From the graph, we see that the values of the two functions at x = 4 are

f(4) = 0

g(4) = 0

Therefore, (f/g)(4) is undefined.