Question

B) Use the quadratic formula to find the roots of each quadratic function. Tell the number of solutions as X = _______

Answer 1

`x\text{ = }(2)/(3)\text{ + i}\frac{\sqrt[]{2}}{3}\text{and x = }(2)/(3)-\text{ i}\frac{\sqrt[]{2}}{3}`

Step-by-step explanation

A quadratic function is generally given as:

`f(x)=ax^2\text{ + bx + c}`

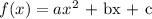
The quadratic formula used to find the roots of a quadratic equation(function) is:

`x\text{ = }\frac{-b\text{ }\pm\text{ }\sqrt[]{b^2\text{ - 4ac}}}{2a}`

From the function given, we have that:

a = 3, b = -4, c = 2

Therefore, the roots of the function are:

`\begin{gathered} x\text{ = }\frac{-(-4)\text{ }\pm\sqrt[]{(-4)^2\text{ - 4(3)(2)}}}{2(3)} \\ x\text{ = }\frac{4\pm\sqrt[]{16\text{ - }24}}{6} \\ x\text{ = }\frac{4\text{ }\pm\sqrt[]{-8}}{6} \\ x\text{ = }\frac{2\text{ + 2 }\sqrt[]{-2}}{3}\text{ and x = }\frac{2\text{ - 2 }\sqrt[]{-2}}{3} \\ x\text{ = }(2)/(3)\text{ + i}\frac{\sqrt[]{2}}{3}\text{and x = }(2)/(3)-\text{ i}\frac{\sqrt[]{2}}{3} \end{gathered}`