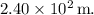Question

Monarch butterflies migrate north during the spring. Suppose on the first day of migration, one butterfly flies east for 180 m and then flies 68 m north before resting on a flower. Then the butterfly goes to the next flower, 2.0x10¹ m to the west, before flying north again for another 231 m. Calculate the magnitude of the butterfly's northeast displacement

Answer 1

The magnitude of the butterfly's northeast displacement is
`\(2.40 * 10^2 \, \text{m}\).`

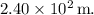
Step-by-step explanation:

The butterfly's displacement involves both eastward and northward components. To find the northeast displacement, we can use the Pythagorean theorem, which states that the magnitude of the resultant displacement
`(\(d\))` is given by
`\(d = √((d_x)^2 + (d_y)^2)\), where \(d_x\)` is the eastward displacement and
`\(d_y\)` is the northward displacement.

In this scenario, the eastward displacement is
`\(180 \, \text{m}\)`(positive because it's to the east) and the northward displacement is

`\(68 \, \text{m}\) minus \(231 \, \text{m}\) (negative because it's southward).`

So,

`\(d_x = 180 \, \text{m}\) and \(d_y = 68 \, \text{m} - 231 \, \text{m} = -163 \, \text{m}\).`

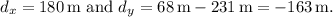
Now, applying the Pythagorean theorem:

`\[ d = \sqrt{(180 \, \text{m})^2 + (-163 \, \text{m})^2} \approx \sqrt{32400 \, \text{m}^2 + 26569 \, \text{m}^2} \approx \sqrt{58969 \, \text{m}^2} \approx 2.40 * 10^2 \, \text{m} \]`

Therefore, the magnitude of the butterfly's northeast displacement is
`\(2.40 * 10^2 \, \text{m}\).`