Question

An oil tank is being filled at a constant rate. The depth of the oil is a function of the number of minutes the tank has been filling, as shown in the table. Find the depth of the oil 45 minutes after filling begins.

Answer 1

12 feet

Step-by-step explanation

We want to find the depth of oil 45 minutes after the filling begins.

To do this, first we have to find an equation that represents the situation (a linear equation).

The general form of a linear equation is:

`\begin{gathered} y=mx+b \\ \text{where m =slope; b = y intercept} \end{gathered}`

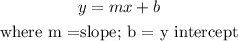
We have to find the slope of the equation.

To do that, we apply the formula for slope:

`\begin{gathered} m=(y2-y1)/(x2-x1) \\ \text{where (x1, y1) and (x2, y2) are two data points from the table} \end{gathered}`

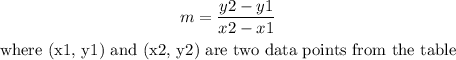
From the equation, x represents minutes and y represents Oil depth.

Let us pick two sets of data points from the table: (0, 3) and (10, 5)

Therefore, slope is:

`\begin{gathered} m=(5-3)/(10-0)=(2)/(10) \\ m=(1)/(5) \end{gathered}`

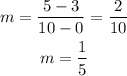
To find the equation, we apply the point-slope method:

`\begin{gathered} y-y1=m(x-x1) \\ \Rightarrow y-3=(1)/(5)(x-0) \\ y-3=(1)/(5)x \\ y=(1)/(5)x+3 \end{gathered}`

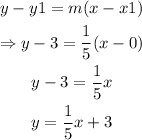
Therefore, to find the depth of oil after 45 minutes, we have to find y when x is 45.

That is:

`\begin{gathered} y=(1)/(5)(45)+3=9+3 \\ y=12\text{ feet} \end{gathered}`

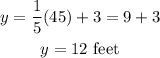
45 minutes after filling begins, the depth of the oil is 12 feet.