Answer:
R = 0.24 kilo-ohm
Step-by-step explanation:
Given that,
Voltage, V = 12 volt
Current, I = 50 mA = 0.05 A
We need to find the resistance of the resistor. We can find it using Ohm's law. So,
V = IR
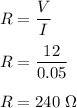
or
R = 0.24 kohm
So, the value of resistance is 0.24 kilo-ohm.