Question

suppose you have a 16 m long piece of copper wire with a 2.362 mm diameter and a resistivity of 1.72 × 10-8 ω⋅m.

Answer 1

The resistance of the copper wire can be calculated using the formula
`\( R = \frac{{\rho \cdot L}}{{A}} \),` where ( R ) is the resistance,
`\( \rho \)` is the resistivity, ( L ) is the length, and ( A ) is the cross-sectional area. In this case, the resistance of the 16 m long copper wire with a 2.362 mm diameter and a resistivity of
`\( 1.72 * 10^(-8) \, \Omega \cdot m \) is approximately \( 0.114 \, \Omega \).`

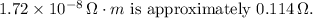
Step-by-step explanation:

To calculate the resistance of the copper wire, we use the formula
`\( R = \frac{{\rho \cdot L}}{{A}} \), where \( R \)` is the resistance,
`\( \rho \)` is the resistivity, ( L ) is the length, and ( A ) is the cross-sectional area. The cross-sectional area ( A ) can be determined using the formula
`\( A = \frac{{\pi \cdot d^2}}{4} \),` where ( d ) is the diameter of the wire.

Given that the diameter of the wire is ( 2.362 ) mm, we convert it to meters by dividing by ( 1000 )
`(since \( 1 \, \text{m} = 1000 \, \text{mm} \)),`giving us a diameter of ( 0.002362 ) m. Plugging this value into the formula for ( A ), we find the cross-sectional area.

Substituting the values of
`\( \rho = 1.72 * 10^(-8) \, \Omega \cdot m \), \( L = 16 \, \text{m} \),` and ( A ) into the formula for resistance, we calculate ( R ) to be approximately
`\( 0.114 \, \Omega \).`