Question

Can some help me with making up a true equation and then I need a false equation.

Answer 1

A true equiation has a real solution, for example

`(3)/(x)+x=(7)/(2)`

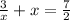
is true equation because you can find the value of x

multiply X to both sides

`\begin{gathered} (3)/(x)(x)+x(x)=(7)/(2)x \\ \end{gathered}`
`\begin{gathered} 3+x^2=(7)/(2)x \\ x^2-(7)/(2)x+3=0 \end{gathered}`

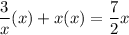
and you can factorize and solve

`\begin{gathered} (x-2)(x-(3)/(2))=0 \\ \end{gathered}`

It has two solutions for x

`\begin{gathered} (x_1-2)=0 \\ x_1=2 \\ (x_2-(3)/(2))=0 \\ x_2=(3)/(2) \end{gathered}`

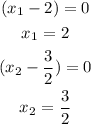
and a false equation has not solution or is not real, for example

`\sqrt[]{x}=-2`

this cant not be, because when you try to solve x you have:

`\begin{gathered} x=(-2)^2 \\ x=4 \end{gathered}`

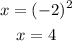
but when you replace to check equality

`\begin{gathered} \sqrt[]{(4)}=-2 \\ 2\\e-2 \end{gathered}`