Question

ketch the bounded region enclosed by y=e^2x,y=e^4x and x=1 . decide whether to integrate with respect to x or y , and then find the area of the region. the area is .

Answer 1

The total area of the regions between the curves is 40477.02 square units

Calculating the total area of the regions between the curves

From the question, we have the following parameters that can be used in our computation:

y = e²ˣ and y = e⁴ˣ

Also, we have that

x = 1

The graph of the region is added as an attachment

And we integrate with respect to x using the following interval

x = 1 and x = 3

So, the area of the regions between the curves is

`\text{Area} = \int\limits^(3)_(1) [e^(4x) - e^(2x)] \, dx`

Integrate

`\text{Area} = \frac{\left(\mathrm{e}^(2x)-2\right)\mathrm{e}^(2x)}{4}`

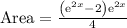
Using the limits, we have

`\text{Area} = \frac{\mathrm{e}^(12)-2\mathrm{e}^6}{4}-\frac{\mathrm{e}^4-2\mathrm{e}^2}{4}`

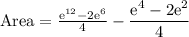
Evaluate

Area = 40477.02

Hence, the total area of the regions between the curves is 40477.02 square units