Question

What value of C will make the following system a dependent system (one in which the lines coincides)15x +20y= -106x + 8y= c

Answer 1

C = -4

Step-by-step explanation

Given:

15x +20y= -10

6x + 8y= c

Thus we have:

a1 = 15x, b1 = 20y, c1 = -10

and

a2 = 6x, b2 = 8y, c2 = c

The condition now is:

`(a_1)/(a_2)\text{ = }(b_1)/(b_2)\text{ = }(c_1)/(c_2)`

i.e:

`(15x)/(6x)=(20y)/(8y)\text{ = }(-10)/(c)`

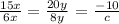
Determine c using the x

`\begin{gathered} (15x)/(6x)=(-10)/(c) \\ (5)/(2)\text{ = }(-10)/(c) \\ 5c\text{ = -20} \\ c\text{ = -}(20)/(5) \\ c\text{ = -4} \end{gathered}`

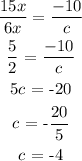
Determine c using the y

`\begin{gathered} (20y)/(8y)\text{ = }(-10)/(c) \\ (10)/(4)\text{ = }(-10)/(c) \\ 10c\text{ = -40} \\ c\text{ = }(-40)/(10) \\ c\text{ = -4} \end{gathered}`

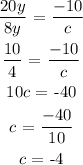
Hence, the value of C that will make the systems a dependent system is -4.