Question

a highway curve of radius 493 m is designed for traffic moving at a speed of 77 km/h. what is the correct banking angle of the road?

Answer 1

The correct banking angle of the road is approximately 16.7 degrees.

The correct banking angle of a road for a given curve is determined by the balance between centripetal force and gravitational force. In this scenario, the centripetal force is provided by the friction between the tires and the road, and the gravitational force acts vertically downward. To find the correct banking angle, one can use the following formula:

tan(θ)= v^2/g⋅r

In this case, with a curve radius of 493 m and a speed of 77 km/h.

​Plugging these values into the formula, we get:

tan(θ)=
`(21.39m/s)^2/9.8m/s^2⋅493m`

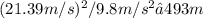
Solving for θ, we find the banking angle is approximately 16.7 degrees. This is the angle at which the road should be banked to ensure vehicles can navigate the curve smoothly without relying solely on friction for the required centripetal force.