Question

Look at points C and D on the graph:What is the distance (in units) between points C and D? Round your answer to the nearest hundredth 4.54 units 34.00 units5.00 units5.83 units

Answer 1

We will investigate how to determine the magnitude ( length ) of a line segment between two points on a cartesian coordinate grid.

We need atleast two points to draw a line segment on a cartesian coordinate grid. The two points C and D are indicated on the given graph. We will go ahead and write down the respective coordinates:

`\begin{gathered} C\colon\text{ ( -4 , 3 )} \\ D\text{ : ( 1 , 0 )} \end{gathered}`

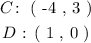
To determine the magnitude or the length of the line segment we use the following formulation:

`|CD|\text{ = }\sqrt[]{(x_2-x_1)^2+(y_2-y_1)^2}`

Where,

`|CD|\text{ : The magnitude of line segment denoted by end points}`

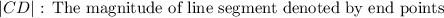
We will use the above distance formula to evaluate the magnitude of any line segment drawn on a cartesian coordinate grid between two points:

`\begin{gathered} C\colon(x_1,y_1)\to\text{ (-4 , 3 )} \\ D\colon(x_2,y_2)\to\text{ ( 1 , 0 )} \end{gathered}`

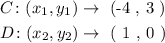
Plug in the respective coordinates of each point in the given formula:

`\begin{gathered} |CD|\text{ = }\sqrt[]{(1-(-4))^2+(0-3)^2} \\ \\ |CD|\text{ = }\sqrt[]{(5)^2+(-3)^2} \\ \\ |CD|\text{ = }\sqrt[]{25+9^{}} \\ \\ |CD|\text{ = }\sqrt[]{34} \\ OR \\ |CD|\text{ = }5.83095\text{ units} \end{gathered}`

We will use the decimal version of the magnitude of line segment. We will be giving our answer to the nearest hundreths i.e the second decimal place. Therefore, the final answer would be:

`5.83\text{ units }\ldots\text{ Option D}`