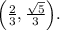Final Answer:
The missing y-coordinate of point P in the second quadrant, lying on the unit circle with x-coordinate
 . Therefore, the coordinates of P are
. Therefore, the coordinates of P are
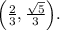
Step-by-step explanation:
In the second quadrant of the Cartesian coordinate system, the x-coordinate is negative, and the y-coordinate is positive. Since P lies on the unit circle, the distance from the origin to P is 1.
Given that the x-coordinate of P is
 , we can use the Pythagorean theorem to find the y-coordinate. The Pythagorean theorem states that for any right-angled triangle, the square of the length of the hypotenuse
, we can use the Pythagorean theorem to find the y-coordinate. The Pythagorean theorem states that for any right-angled triangle, the square of the length of the hypotenuse
 is equal to the sum of the squares of the lengths of the other two sides
is equal to the sum of the squares of the lengths of the other two sides
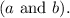
In this case, let (a) be the x-coordinate
 , (b) be the y-coordinate, and (c) be the distance from the origin to P (which is 1 on the unit circle).
, (b) be the y-coordinate, and (c) be the distance from the origin to P (which is 1 on the unit circle).
The Pythagorean theorem equation is:
![\[ a^2 + b^2 = c^2 \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/ztlj7w2crnu8irtu4w20tft65vmrapasq9.png)
Substitute the given values:
![\[ \left((2)/(3)\right)^2 + b^2 = 1^2 \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/sayd1tj45e6hq1g58t5lfgvvcgnkmvo6im.png)
![\[ (4)/(9) + b^2 = 1 \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/aqgz0minqsg3bp8xvbl9okypd39utrxvz0.png)
Now, solve for \(b\):
![\[ b^2 = 1 - (4)/(9) \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/c9iks5x0fw73c3zwznr6ozhg7rtvd2v3tb.png)
![\[ b^2 = (5)/(9) \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/sfa2iopgwqfysqbxzidni7lgwznlq0e0a1.png)
![\[ b = \pm \sqrt{(5)/(9)} \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/yqquupc359e2hv7fpmvr6ef6xmnns64ks8.png)
Since P is in the second quadrant where the y-coordinate is positive, the y-coordinate of P is
 .
.
Therefore, the coordinates of P in the second quadrant are