Question

For 1-12, use partial quotients to divide.

You may use counters or draw pictures to help.
1.4)92

Answer 1

Partial quotients involve breaking down a square root expression into parts to simplify the division. Let's go through the process for each:

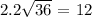
`1. \(4√(92)\) = 8√(23)`

`2. \(2√(36)\) = 12`

3.
`\(5√(76)\) = 10√(19)`

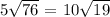
4.
`\(3√(72)\) = 6√(18)`

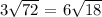
5.
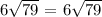
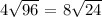
`\(6√(79)\) = 6√(79)`

6.
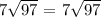
`\(4√(96)\) = 8√(24)`

7.
`\(7√(97)\) = 7√(97)`

8.
`\(3√(99)\) = 9√(11)`

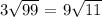
Partial quotients involve breaking down a square root expression into parts to simplify the division. Let's go through the process for each:

`1. \(4√(92)\) 4√(92) = 4√(4 * 23) = 8√(23)\]\\2. \(2√(36)\) 2√(36) = 2 * 6 = 12\]\\3. \(5√(76)\) 5√(76) = 5√(4 * 19) = 10√(19)\]\\4. \(3√(72)\) 3√(72) = 3√(4 * 18) = 6√(18)\]\\`

`5. \(6√(79)\) 6√(79)\] (No perfect square factors, so it remains in radical form)\\6. \(4√(96)\) 4√(96) = 4√(4 * 24) = 8√(24)\]\\7. \(7√(97)\) 7√(97)\] (No perfect square factors, so it remains in radical form)\\8. \(3√(99)\) 3√(99) = 3√(9 * 11) = 9√(11)\]`

These results represent the simplified forms of the given square root expressions using partial quotients.