Question

(5 points each part) Write an exponential equation and use logs to solve.a. The population of rabbits on a rabbit farm increases by 250% eachyear. The farm started its business with 12 rabbits. How long will ittake the farm’s rabbit population to grow to 250 rabbits?

Answer 1

The form of the exponential equation is

`y=a(1+r)^x`

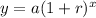
a is the initial amount

r is the annual rate in decimal

Since the number of rabbits increases by 250% each year, then

`r=(250)/(100)=2.5`

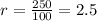
Since the farm started with 12 rabbits, then

`a=12`

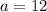
Substitute them in the form of the equation above

`\begin{gathered} y=12(1+2.5)^x \\ y=12(3.5)^x \end{gathered}`

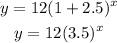
We need to find the time for the rabbits to be 250

Then substitute y by 250 and solve the equation to find x

`250=12(3.5)^x`

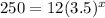
Divide both sides by 12

`\begin{gathered} (250)/(12)=(12(3.5)^x)/(12) \\ (125)/(6)=(3.5)^x \end{gathered}`

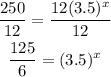
Insert log to both sides

`\log ((125)/(6))=\log (3.5)^x`

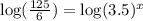
Use the rule of the exponent with log

`\log (a)^n=n\log (a)`
`\log ((125)/(6))=x\log (3.5)`

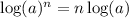
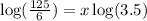
Divide both sides by log(3.5) to find x

`\begin{gathered} (\log((125)/(6)))/(\log(3.5))=(x\log (3.5))/(\log (3.5)) \\ 2.423885719=x \end{gathered}`

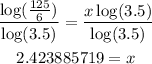
It will take about 2.42 years to be 250 rabbits