Answer:
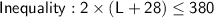
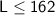
Explanation:
Let's denote the length of the rectangle as
 . The perimeter (
. The perimeter (
 ) of a rectangle is given by the formula:
) of a rectangle is given by the formula:
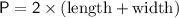
In this case, the width (
 ) is given as 28 centimeters, and the perimeter should be no more than 380 centimeters.
) is given as 28 centimeters, and the perimeter should be no more than 380 centimeters.
So, the inequality representing the perimeter constraint is:
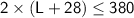
Now, let's solve for
 :
:
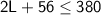
Subtract 56 from both sides:
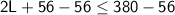

Divide by 2 on both sides:
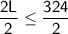
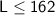
So, the possible lengths of the rectangle (
 ) should be less than or equal to 162 centimeters to ensure that the perimeter is no more than 380 centimeters
) should be less than or equal to 162 centimeters to ensure that the perimeter is no more than 380 centimeters