Question

Line m has the equation 2x + 3y = 6, line n passes through the points in the table, and line p has the graph shown in the figure. Which of these lines, if any, are perpendicular? Explain.

Answer 1

The general equation of line passing through a point (x, y) can be written as,

`y=mx+b`

Here, m is the slope and b is the y intercept. Th slope can be calculated as,

`m=(y2-y1)/(x2-x1)`

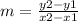
For two lines to be perpendicular, the slopes will be reciprocal two each other and in opposite sign.

The given lines m is,

`m\rightarrow2x+3y=6\rightarrow y=-(2)/(3)x+2`

The slope is therefore,

`m\rightarrow-(2)/(3)`

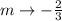
Now, the slope of the line n can be calculated as,

`n\rightarrow(11-8)/(8-6)=(3)/(2)`

The slope for the line p passing through the pointes (0,3) (3, 4)can be calculated as,

`p\rightarrow(4-3)/(3-0)=(1)/(3)`

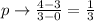
From the calculated slopes, we can infer that the lines m and n are perpendicular to each other.