First, we can notice that all the optins have the same x values: 0, 1, 2 and 3.
These are at equal differences of 1.
A quadratic relationship has the property that the difference of the differences of subsequent points is constant.
So, to check wheter it is a quadratic relationship, we can calculate the differences between f(1) and f(0), f(2) and f(1) and f(3) and f(2):
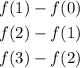
And then we make the difference between the first and second differences and between the third and second. if they are equal and difference than 0, it is a quadratic relationship.
For the first, we have:
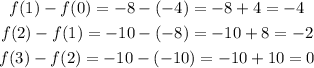
Now, the difference of differences:
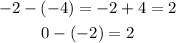
They are equal, so the first one is a quadratic relationship.
The second, we have:
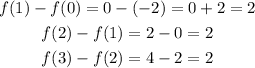
Now, the difference of differences:
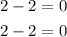
They are the same but they are equal to zero, so the second is not a quadratic relationship. This will happen when the first differences are constant, becaise this is a linear relationship. Notice that the fifth also have constant differences, so the fifth is not a quadratic relationship.
For the third, we have:
e have:
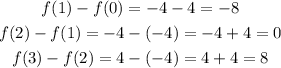
Now, the difference of differences:
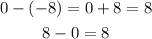
They are equal so, the third is also a quadratic relationship.