Final Answer:
A right triangle has side lengths ac = 7 inches, bc = 24 inches, and ab = 25 inches.Measures of the angles in triangle abc are 3)

Step-by-step explanation:
In a right triangle, the angles can be determined using trigonometric ratios. Given the side lengths
 , where
, where
 is the hypotenuse, the cosine of angle
is the hypotenuse, the cosine of angle
 s given by
s given by
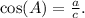
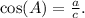
Using the inverse cosine function, we can find the measure of angle
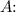
![\[ A = \cos^(-1)\left((7)/(25)\right) \approx 73.7° \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/bixjbwhhkueilq6kgoykouuh4an89ot89m.png)
Similarly, angle
 can be found using the sine ratio:
can be found using the sine ratio:
![\[ \sin(B) = (a)/(c) \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/wjtwju30b5lrt36ylk5u1qvg9fgoa8acv0.png)
![\[ B = \sin^(-1)\left((7)/(25)\right) \approx 16.3° \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/dgvlkq8pozeiaynbxzsru66jc2x1j3ev79.png)
Since it is a right triangle, the sum of angles
 should equal
should equal
 making angle
making angle
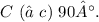
Therefore, the correct answer is option 3, where
 and
and
 This corresponds to the angles calculated using trigonometric ratios in a right triangle with side lengths
This corresponds to the angles calculated using trigonometric ratios in a right triangle with side lengths
 inches.
inches.