Question

The mean height of an adult giraffe is 18 feet. Suppose that the distribution is normally distributed with standard deviation 1 feet. Let X be the height of a randomly selected adult giraffe. Round all answers to 4 decimal places where possible.a. What is the distribution of X? X ~ N(18Correct,1Correct) b. What is the median giraffe height? 18Correct ft. c. What is the Z-score for a giraffe that is 21 foot tall? 1.5Incorrectd. What is the probability that a randomly selected giraffe will be shorter than 18.6 feet tall? 0.6Incorrecte. What is the probability that a randomly selected giraffe will be between 17.2 and 18 feet tall? 1.042Incorrectf. The 80th percentile for the height of giraffes is 18.5844Incorrect ft.

Answer 1

Question C:

- The formula for the Z-score is given below:

`\begin{gathered} Z=(X-\mu)/(\sigma) \\ where, \\ \mu=\text{ mean} \\ \sigma=\text{ Standard deviation} \end{gathered}`

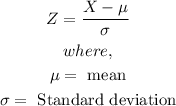
- Thus, we can calculate the Z-score as follows:

`\begin{gathered} \mu=18,\sigma=1,X=21 \\ \\ \therefore Z=(21-18)/(1) \\ \\ Z=3 \end{gathered}`

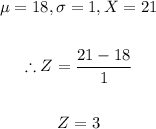
- The Z-score is 3

Question D:

- We need to calculate the Z-score of the height of 18.6ft and then convert the Z-score to probability using a Z-score calculator.

- The Z-score is calculated as follows:

`\begin{gathered} X=18.6 \\ Z=(18.6-18)/(1) \\ Z=0.6 \end{gathered}`

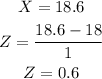
- The probability P(Z < 0.6) is depicted below:

- Thus, the probability of getting a giraffe shorter than 18.6ft is 0.72575

Question E:

- Again, we need to find the Z-scores for both 17.2ft and 18ft and then find the probability:

P(17.2 < Z < 18)

- The Z-scores are gotten as follows:

`\begin{gathered} Z_1=(17.2-18)/(1) \\ Z_1=-0.8 \\ \\ Z_2=(18-18)/(1) \\ Z_2=0 \end{gathered}`

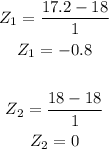
- Thus, the probability of obtaining heights corresponding to the above Z-scores is:

- Thus, the probability of getting a giraffe with a height between 17.2 and 18ft tall is 0.28814

Question F:

- The 80th percentile corresponds to 80/100 = 0.8 probability.

- We should find the corresponding Z-score before finding the value of X, using the Z-score formula

- The corresponding Z-score of 0.8 is 0.842

- The value of X is:

`\begin{gathered} Z=(X-\mu)/(\sigma) \\ \\ 0.842=(X-18)/(1) \\ \\ Add\text{ 18 to both sides} \\ \\ \therefore X=18+0.842 \\ \\ X=18.842 \end{gathered}`

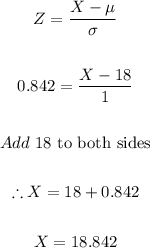
- The 80th percentile value is 18.842