Question

what are the solutions to the system of equations
`y = (x + 4){2} - 1`and
`y = - 3 - 13`

Answer 1

We are given the following two equations

`\begin{gathered} y=(x+4)^2-1\qquad eq.1_{} \\ y=-3x-13\qquad eq.2 \end{gathered}`

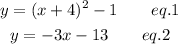
Since both equations are equal to y then we can equate them as

`(x+4)^2-1=-3x-13`

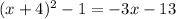
Now let us simplify the equation

`\begin{gathered} (x+4)^2-1=-3x-13 \\ x^2+2(x)(4)+4^2-1=-3x-13 \\ x^2+8x+16-1=-3x-13 \\ x^2+8x+3x+16-1+13=0 \\ x^2+11x+28=0 \end{gathered}`

As you can see, we are left with a quadratic equation.

The standard form of a quadratic equation is given by

`ax^2+bx+c=0`

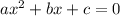
Comparing the equation with the standard form, the coefficients are

a = 1

b = 11

c = 28

Now recall that the quadratic formula is given by

`x=(-b\pm√(b^2-4ac))/(2a)`

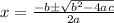
Substitute the values into the above quadratic formula

`x=\frac{-11\pm\sqrt[]{(11)^2-4(1)(28)}}{2(1)}=\frac{-11\pm\sqrt[]{121^{}-112}}{2}=\frac{-11\pm\sqrt[]{9}}{2}=(-11\pm3)/(2)`

So the two possible solutions are

`\begin{gathered} x_1=(-11+3)/(2),\: x_2=(-11-3)/(2) \\ x_1=(-8)/(2),\: x_2=(-14)/(2) \\ x_1=-4,\: x_2=-7 \end{gathered}`

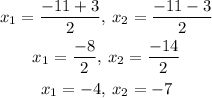
Therefore, the solutions of the equations are

x = -4 and x = -7