Question

(d) Determine the maximum number of turning points on the graph of the function.

Answer 1

Given the following function

`f(x)=x^2(x+10)(x-10)`

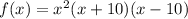
We want to know the maximum number of turning points of this function. To determinate the maximum number of turning points, first we need to understand what is a turning point.

A turning point is is a point where the first derivative is null.

To find the turning points then, we just need to calculate the first derivative and solve for f'(x) = 0.

To find the derivative, we can just expand the polynomial and use the power rule.

`x^2(x+10)(x-10)=x^2(x^2-100)=x^4-100x^2_{}`
`f^(\prime)(x)=4x^3-200x`

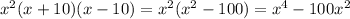
Now, we just need to solve for f'(x) = 0 to find the turning points, but, since we only want to know the maximum amount of turning points is even easier to find. This is a third-degree polynomial, and the solutions for the f'(x) = 0 are the roots of this polynomial. Since it is a third-degree polynomial, the maximum amount of roots is 3.

From the statements of item C, we can see the correct graph is the graph C.