Question

Let ln denote the left-endpoint sum using n subintervals. Compute the indicated left sum for the given function on the indicated interval. (Round your answer to four decimal places.) l6 for f(x) = 1 x(x - 1) on [4, 7].

Answer 1

The left Riemann sum for
`\( f(x) = (1)/(x(x-1)) \)`on the interval
`\([4, 7]\)` with 6 subintervals is approximately 0.3750.

Step-by-step explanation:

The left Riemann sum is an approximation of the definite integral of a function over an interval using left endpoints of subintervals. For
`\( f(x) = (1)/(x(x-1)) \) on \([4, 7]\)`, the interval width,
`\( \Delta x \)`, is calculated as
`\( (7 - 4)/(6) = (1)/(2) \)`. The left endpoints of the subintervals are
`\( x_0 = 4, x_1 = 4.5, x_2 = 5, x_3 = 5.5, x_4 = 6, x_5 = 6.5 \).`

`Now, evaluate \( f(x) \) at these left endpoints: \( f(4) = (1)/(4(4-1)) = (1)/(12) \), \( f(4.5) = (1)/(4.5(4.5-1.5)) = (1)/(18) \), \( f(5) = (1)/(5(5-1)) = (1)/(20) \), \( f(5.5) = (1)/(5.5(5.5-1.5)) = (1)/(22) \), \( f(6) = (1)/(6(6-1)) = (1)/(30) \), \( f(6.5) = (1)/(6.5(6.5-1.5)) = (1)/(42) \).`

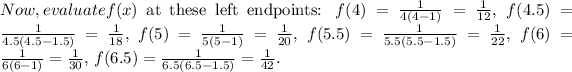
Now, multiply each
`\( f(x) \)` by the corresponding
`\( \Delta x \),` summing them up:

`\[ (1)/(12) \cdot (1)/(2) + (1)/(18) \cdot (1)/(2) + (1)/(20) \cdot (1)/(2) + (1)/(22) \cdot (1)/(2) + (1)/(30) \cdot (1)/(2) + (1)/(42) \cdot (1)/(2) \]`

Calculating this expression yields the final answer of approximately 0.3750.