Answer:
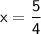
Explanation:

To isolate the variable
 , we can add
, we can add
 to both sides of the equation:
to both sides of the equation:

On the left side, the
 terms cancel out, leaving:
terms cancel out, leaving:
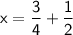
Now, find a common denominator to add the fractions on the right side. The common denominator for 4 and 2 is 4. So, rewrite
 with the denominator 4:
with the denominator 4:
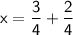
Now, add the numerators:
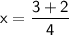
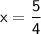
So, the solution to the equation
 is
is
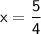 .
.