Question

Rick paddled up the river, spent the night camping, and then paddled back. He spent 14 hours paddling, and the campground was 52 miles away. If Rick kayaked at a speed of 8 miles per hour, what was the speed of the current?

Answer 1

Given:

Number of hours, t = 14 hours

Distance = 52 miles

Speed travelling = 8 mph

Let's find the speed of the current.

Apply the formula:

`\begin{gathered} \text{ speed=}(distnace)/(time) \\ \\ time=(distance)/(speed) \end{gathered}`

Here, we have the system of equations:

For time travelling up: 14 = 52/8-c

For time travelling down: 14 = 52/8 + c

Where c is the speed of the current.

Hence, we have:

`(52)/(8-c)+(52)/(8+c)=14`

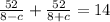
Let's solve the equation for c.

Multiply all terms by (8-c)(8+c):

`\begin{gathered} (52)/(8-c)(8-c)(8+c)+(52)/(8+c)(8-c)(8+c)=14(8-c)(c+c) \\ \\ 52(8+c)+52(8-c)=14(8-c)(8+c) \end{gathered}`

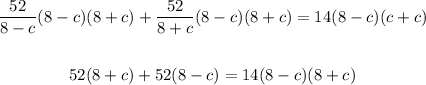
Solving further, expand using FOIL method and apply distributive property:

`\begin{gathered} 52(8)+52c+52(8)-52c=14(64-c^2) \\ \\ 416+52c+416-52c=896-14c^2 \\ \\ 416+416+52c-52c=896-14c^2 \\ \\ 832-896=-14c^2 \\ \\ -64=-14c^2 \end{gathered}`

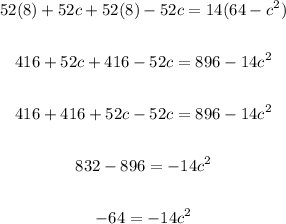
Solving further:

Divide both sides by -14

`\begin{gathered} (-64)/(-14)=(-14c^2)/(-14) \\ \\ 4.57=c^2 \\ \\ c^2=4.57 \\ \\ \text{ Take the square root of both sides:} \\ √(c^2)=√(4.57) \\ \\ c=2.14 \end{gathered}`

Therefore, the speed of the current was 2.14 miles per hour.

ANSWER:

2.14 mph