Question

Shown below in these images are my calculus questions. Any help you give is much appreciated, I'm trying to catch up for end of term.

Answer 1

`\textsf{1)}\quad (d)/(dx)\left(3^x\right)=\boxed{3^x\ln 3}`

`\begin{aligned}\textsf{2)}\quad &f'(x)=\boxed{(12)/(x)}\\&f'(8)=\boxed{(3)/(2)}\\&f''(x)=\boxed{-(12)/(x^2)}\\&f''(8)=\boxed{-(3)/(16)}\end{aligned}`

`\textsf{3)} \quad (d)/(dx)\left(4 \log_2(x)+13\right)=\boxed{(4)/(x \ln(2))}`

`\textsf{4)} \quad f'(x)=\boxed{(9)/(x)+(5)/(x+3)+(14x)/(x^2+5)}`

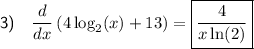
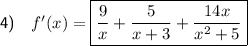
Explanation:

Question 1

To find the derivative of 3^x we can use the following rule:

`\boxed{\begin{array}{c} \underline{\textsf{Differentiating $a^x$}}\\\\(d)/(dx)\left(a^x\right)=a^x \ln (a)\\\\\textsf{for any constant $a$}\end{array}}`

Therefore:

`(d)/(dx)\left(3^x\right)=3^x\ln (3)`

`\hrulefill`

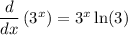
Question 2

To differentiate f(x) = 12 ln(x), we can use the differentiation rule for ln(x):

`\boxed{\begin{array}{c} \underline{\textsf{Differentiating $\ln(x)$}}\\\\(d)/(dx)\left(\ln(x)\right)=(1)/(x)\end{array}}`

First, take the constant out:

`(d)/(dx)\left(12 \ln(x)\right)=12 \cdot (d)/(dx)\left(\ln(x)\right)`

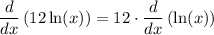
Now, apply the derivative rule:

`\begin{aligned}&=12 \cdot (1)/(x)\\\\&=(12)/(x)\end{aligned}`

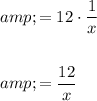
Therefore:

`f'(x)=(12)/(x)`

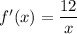
To find f'(8), simply substitute x = 8 into f'(x):

`f'(8)=(12)/(8)=(3)/(2)`

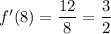
To find the second derivative f''(x), we can differentiate f'(x).

Begin by rewriting the denominator of f'(x) using the negative exponent rule:

`f'(x)=(12)/(x)=12x^(-1)`

Now, use the power rule for differentiation:

`\boxed{\begin{array}{c} \underline{\textsf{Power Rule}}\\\\(d)/(dx)\left(x^n\right)=nx^(n-1)\end{array}}`

Therefore:

`f''(x)=(-1) \cdot 12x^(-1-1)`

`f''(x)=-12x^(-2)`

`f''(x)=-(12)/(x^2)`

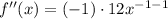
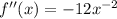
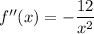
To find f''(8), substitute x = 8 into f''(x):

`f''(x)=-(12)/(8^2)=-(12)/(64)=-(3)/(16)`

`\hrulefill`

Question 3

To differentiate 4log₂(x) + 13, we can use the derivative log rule and the the constant rule:

`\boxed{\begin{array}c \begin{array}{c}\underline{\textsf{Log Rule}}\\\\ (d)/(dx)\left(\log_a(x)\right)=(1)/(x\ln(a))\\\\\textsf{for any constant $a$}\end{array}&\begin{array}{c}\underline{\textsf{Constant Rule}}\\\\(d)/(dx)(a)=0\\\\\textsf{for any constant $a$}\end{array}\end{array}}`

Therefore:

`\begin{aligned}(d)/(dx)\left(4 \log_2(x)+13\right)&=4 \cdot (1)/(x \ln(2))+0\\\\&=(4)/(x \ln(2))\end{aligned}`

`\hrulefill`

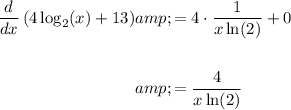
Question 4

Given:

`f(x)=\ln \left[x^9(x+3)^5(x^2+5)^7\right]`

Before taking the derivative of f(x), we can expand the function using the Properties of Logarithms.

`\boxed{\begin{array}{rl}&\underline{\sf Logarithmic\;Properties}\\\\\sf Product\;Rule:&\ln(xy)=\ln(x)+\ln(y)\\\\\sf Power\;Rule:&\ln(x^n)=n\ln(x)\\\\\end{array}}`

First, apply the product rule:

`f(x)=\ln (x^9)+\ln ((x+3)^5)+\ln ((x^2+5)^7)`

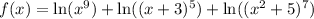
Then, apply the power rule:

`f(x)=9\ln (x)+5\ln (x+3)+7\ln (x^2+5)`

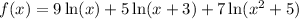
Now we can differentiate f(x) using the following rule:

`\boxed{\begin{array}{c} \underline{\textsf{Differentiating $\ln(f(x))$}}\\\\(d)/(dx)\left(\ln(f(x))\right)=(f'(x))/(f(x))\end{array}}`

Therefore:

`\begin{aligned}(d)/(dx)\left[9\ln (x)+5\ln (x+3)+7\ln (x^2+5)\right]&=9 \cdot (1)/(x)+5 \cdot (1)/(x+3)+7 \cdot (2x)/(x^2+5)\\\\&=(9)/(x)+(5)/(x+3)+(14x)/(x^2+5)\end{aligned}`