Question

A scientist has two solutions, which she has labeled Solution A and Solution B. Each contains salt. She knows that Solution A is 40% salt and Solution B is 65% salt. She wants to obtain 150 ounces of a mixture that is 55% salt. How many ounces of each solution should she use

Answer 1

Since she wants to obtain 150 ounces of the mixture of A and B, then

Add A and B, then equate the sum by 150

`A+B=150\rightarrow(1)`

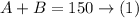
Since 40% of A is salt

Since 65% of B is salt

Since 150 ounces has 55% salt, then

`\begin{gathered} (40)/(100)A+(65)/(100)B=(55)/(100)(150) \\ \\ 0.40A+0.65B=82.5\rightarrow(2) \end{gathered}`

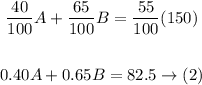
Now, we have a system of equations to solve it

Multiply equation (1) by -0.40 to make the coefficients of A equal in values and opposite in signs

`-0.40A-0.40B=60\rightarrow(3)`

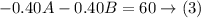
Add equations (2) and (3)

`\begin{gathered} (0.40A-0.40A)+(0.65B-0.40B)=(82.5-60) \\ \\ 0+0.25B=22.5 \\ \\ 0.25B=22.5 \end{gathered}`

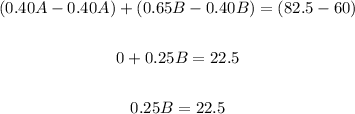
Divide both sides by 0.25

`\begin{gathered} (0.25B)/(0.25)=(22.5)/(0.25) \\ \\ B=90 \end{gathered}`

Substitute B in equation (1) by 90 to find A

`A+90=150`

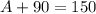
Subtract 90 from each side

`\begin{gathered} A+90-90=150-90 \\ \\ A=60 \end{gathered}`

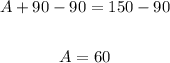
There are 60 ounces of A and 90 ounces of B