Question

I need help with this practice problem I have attempted this and I’ll show you a picture later of it

Answer 1

`(50440)/(729)`

Step-by-step explanation

A geometric serie has a common ratio, the formula for the nth term is

`\begin{gathered} a_n=a\cdot r^(n-1) \\ where \\ a_n=n^(th)\text{ term of the sequ}ence \\ a=\text{ first term of the sequence} \\ r\text{ is the common ratio} \end{gathered}`

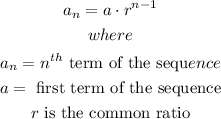
so

Step 1

find the geometric serie

a) find the common ratio

let

`\begin{gathered} \text{first term= 120, so} \\ a_n=a\cdot r^(n-1) \\ 120=a\cdot r^(1-1) \\ 120=a\cdot r^0=a\cdot1 \\ 120=a \end{gathered}`

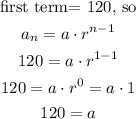
so

a=120

and

`\begin{gathered} \text{second term} \\ -80=a\cdot r^(2-1) \\ -80=120\cdot r^1 \\ -(80)/(120)=r \\ r=-(2)/(3) \\ \text{hence} \\ a_n=\text{ 120(}(-2)/(3))^(n-1) \end{gathered}`

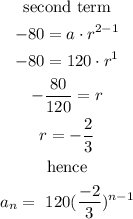
so, the sequence is

`a_n=\text{ 120(}(-2)/(3))^(n-1)`

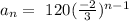
let's check

`\begin{gathered} a_n=\text{ 120(}(-2)/(3))^(n-1) \\ a=1 \\ a_1=\text{ 120(}(-2)/(3))^(1-1)=\text{120(}(-2)/(3))^0=120\rightarrow ok \\ a=2 \\ a_2=\text{120(}(-2)/(3))^(2-1)=120\cdot(-(2)/(3))=-80\rightarrow ok \\ a=3 \\ a_3=\text{ 120(}(-2)/(3))^(3-1)=120((-2)/(3))^2=53.33=(160)/(3)\rightarrow ok \\ a=\text{ 4} \\ a_4=\text{ 120(}(-2)/(3))^(4-1)=\text{120(}(-2)/(3))^3=-35.55\rightarrow ok \end{gathered}`

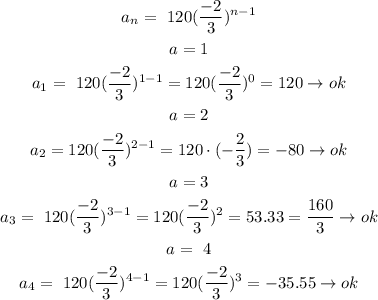
Step 2

now, let's find the sum:

the sum of geometric serie is give by

`\begin{gathered} S_n=(a(1-r^n))/(1-r) \\ \end{gathered}`

let n= 8,

replace

`\begin{gathered} S_n=(120(1-(-(2)/(3))^8))/(1-(-(2)/(3))) \\ S_n=(120(1-(-(2)/(3))^8))/(1+(2)/(3)) \\ S_n=\frac{120(1-((256)/(6561))^{})}{(5)/(3)} \\ S_n=\frac{120(1-((256)/(6561))^{})}{(5)/(3)} \\ S_n=(120((6305)/(6561)))/((5)/(3)) \\ S_n=(120((6305)/(6561)))/((5)/(3))=(120\cdot6305\cdot3)/(6561\cdot5)=(2269800)/(32805)=(50440)/(729) \end{gathered}`

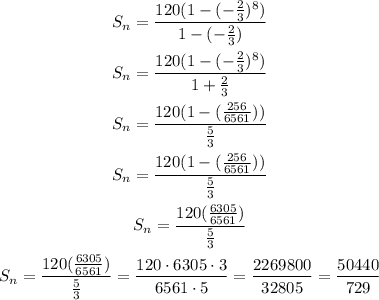
therefore, the answer is

`(50440)/(729)`

I hope this helps you