The amount owed after four years, assuming continuous compounding, is approximately $3357.65.
To solve this problem
We can use the formula for continuous compound interest:
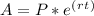
Where:
- A is the total debt after t years.
- P is equal to the principal, or the whole amount borrowed.
- e is equal to Euler's number, or around 2.71828.
- r is the annual interest rate (given in decimal notation).
- t = the total years
Given:
P = $3100
r = 0.02 (2% expressed as a decimal)
t = 4 years
When we enter the provided values into the formula, we get:
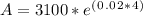
Now, we can calculate the value of
 utilizing a calculator. The result is approximately 1.082085.
utilizing a calculator. The result is approximately 1.082085.
Reentering this value into the formula yields the following:
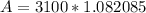
Now, calculating this, we find:
A ≈ $3,357.65
Therefore, the amount owed after four years, assuming continuous compounding, is approximately $3357.65.