Question

What is the total surface area of the prism below?8 cm12 cm.6 cm.

Answer 1

Given:

Required:

To find the total surface area of the prism.

Step-by-step explanation:

The total surface area of the prism is given by the formula:

`A=2* base\text{ area+Area of the rectangle}`

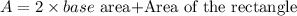
Area of the base triangle

`\begin{gathered} =(1)/(2)* base* height \\ =(1)/(2)*6*8 \\ =(48)/(2) \\ =24\text{ cm}^2 \end{gathered}`

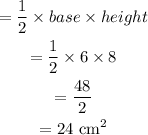
To find the width of the rectangle we will use the Pythagoras theorem.

`\begin{gathered} width=√((8)^2+(6)^2) \\ width=√(64+36) \\ width=√(100) \\ width=10\text{ cm} \end{gathered}`

Area of the rectangle

`\begin{gathered} =length* breadth \\ =12*10 \\ =120cm^2 \end{gathered}`

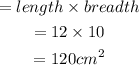
Thus the area of the prism

`\begin{gathered} =2*24+120 \\ =48+120 \\ =168\text{ cm}^2 \end{gathered}`

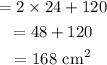
Final Answer: