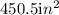Question

Find the area of the figure (area of the shaded region)

Answer 1

`\begin{equation*} 450.5\mathrm{i}n^2 \end{equation*}`

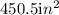
Step-by-step explanation:

Step 1. The first step is to find the hypotenuse of the right triangle, which will also be the diameter of the circle.

To find the missing length of the triangle, we use the Pythagorean theorem:

Where a and b are the legs of the triangle and c is the hypotenuse.

In this case:

a=20in

b=21in

Let d be the diameter of the circle and the hypotenuse of the triangle:

Step 2. Using the Pythagorean theorem to find d:

`\begin{gathered} d^2=20^2+21^2 \\ d^2=400+441 \\ d^2=841 \\ d=√(841) \\ d=29in \end{gathered}`

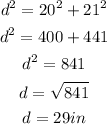
Step 3. Now that we know the value of d, we can find the radius r of the circle:

`\begin{gathered} r=d/2 \\ r=29in/2 \\ r=14.5in \end{gathered}`

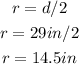
Step 4. To find the area of the shaded region, we need to subtract the triangle's area from the circle's area.

Area of the circle:

`A_(circle)=\pi r^2`

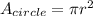
Substituting the value of r:

`\begin{gathered} A_(c\imaginaryI rcle)=\pi(14.5in)^2 \\ A_(c\imaginaryI rcle)=\pi*210.25\imaginaryI n^2 \\ Using\text{ } \\ \pi=3.1416 \\ A_(c\imaginaryI rcle)=3.1416*210.25\imaginaryI n^2 \\ A_(c\imaginaryI rcle)=660.5214\imaginaryI n^2 \end{gathered}`

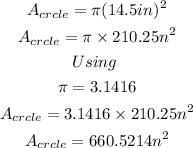
Step 5. Finding the area of the triangle:

`\begin{gathered} A_(triangle)=(b* h)/(2) \\ where\text{ } \\ b=21in \\ h=20in \end{gathered}`

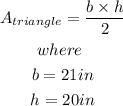
The result is:

`\begin{gathered} A_(triangle)=(21in*20in)/(2) \\ A_(tr\imaginaryI angle)=(420\imaginaryI n^2)/(2) \\ A_(tr\imaginaryI angle)=210\imaginaryI n^2 \end{gathered}`

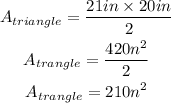
Step 6. To find the area of the shaded region, subtract from the area of the circle the area of the triangle=

`A_(shaded)=A_(circle)-A_(triangle)`

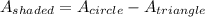
The result is:

`\begin{gathered} A_(shaded)=660.5214\mathrm{i}n^2-210in^2 \\ \boxed{A_(shaded)=450.5214in^2} \end{gathered}`

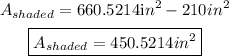
Rounding to the nearest tenth:

`\boxed{A_(shaded)=450.5\imaginaryI n^2}`

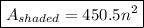
Answer:

`\begin{equation*} 450.5\mathrm{i}n^2 \end{equation*}`