Question

The area of a parallelogram is given by the formula A = bh.

The area of the parallelogram on the right is 65 sq. units.
Find its base and height.
3x+1
The base of the parallelogram is units. (Type an integer or a simplified fraction.)

Answer 1

The base of the parallelogram is equal to 13 units.

The height of the parallelogram is equal to 5 units.

In Mathematics and Euclidean Geometry, the area of a parallelogram can be calculated by using the following formula:

Area of a parallelogram, A = b × h

Where:

• b represents the base area of a parallelogram.
• h represents the height of a parallelogram.

By substituting the given parameters into the formula for the area of a parallelogram, we have the following;

65 = (3x + 1)(x + 1)

`65=3x^2+3x+x+1\\\\65=3x^2+4x+1\\\\3x^2+4x-64=0\\\\(3x+16)(x-4)=0`

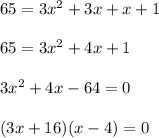
x = 4 or x = -16/3

Since the length cannot assume a negative value, x must be 4 units.

For the base and height, we have;

b = 3x + 1

b = 3(4) + 1

b = 13 units.

h = x + 1

h = 4 + 1

h = 5 units.

Complete Question:

The area of a parallelogram is given by the formula Upper A equals bh. A=bh. The area of the parallelogram on the right is 65 sq. units. Find its base and height.

A parallelogram that has a base labeled 3x + 1 and a height labeled x + 1.