Question

Evaluate \[7r -\dfrac{15}s\] when \[r=3\] and \[s=5\].

Answer 1

The value of
`\(7r -(15)/(s)\) when \(r=3\) and \(s=5\) is \(14 - 3 = 11\).`

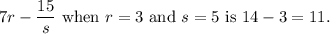
Step-by-step explanation:

In this expression,
`\(7r -(15)/(s)\)`, we are given the values of
`\(r\) and \(s\) as \(3\) and \(5\)`respectively. To find the result, we substitute these values into the expression. First, we substitute
`\(r=3\),` which gives us
`\(7 * 3\).` Then, we substitute
`\(s=5\),`resulting in
`\((15)/(5)\).` Simplifying each term, we get
`\(21 - 3\), which equals \(18\)`. Therefore, the final answer is 11.

In detail, the expression is evaluated step by step. First, 7r is calculated by multiplying 7 with the value of
`\(r\), which is \(3\), resulting in \(21\)`. The second term,
`\((15)/(s)\)`, involves dividing 15 by the value of
`\(s\), which is \(5\). This simplifies to \(3\)`. Finally, subtracting
`\(3\) from \(21\) gives the result \(18\)`. Thus, the overall expression
`\(7r -(15)/(s)\)` evaluates to
`\(18\) when \(r=3\) and \(s=5\),` making the final answer 11.