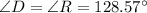Complete Question:
The deck that Amos is building is in the shape of a parallelogram, DGRY. The measure of D is five-halves the measure of Y. Find the measure of each angle of the deck.
Answer:
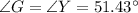
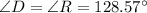
Explanation:
Given
Shape: Parallelogram DGRY
Dimension:
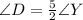
Required
Find the measure of each angle
D and R are opposite sides & G and Y are also opposite sides.
So, we have:
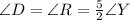
and
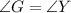
The sum of angles is given as:
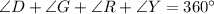
Substitute values for
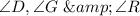
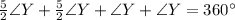
Take LCM
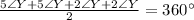

Multiply both sides by
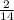
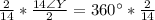
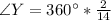
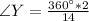
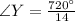
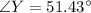
So:
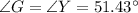
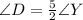
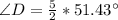
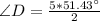
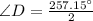
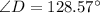
Hence: