Given
m = 20 kg
h = 100m
Kinetic energy and potential energy after
(i) 1 sec
(ii) 2 sec
Procedure
The motion we are analyzing is a free fall, therefore the acceleration faced by the object is 9.8m/s^2 which is gravity.
Kinetic energy is a form of energy that an object or a particle has by reason of its motion.
Let's first calculate the velocity after 1s
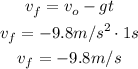
Now with the velocity, we can calculate the kinetic energy
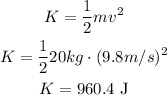
Now let's calculate the potential energy after 1s. Let's first calculate the displacement after that time

Now let us proceed to calculate the potential energy
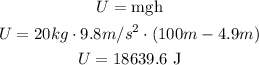
For 1s:
K = 960.4 J
U = 18639.6 J
In a very similar way we are going to calculate for the second 2
Let's first calculate the velocity after 2s
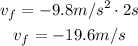
Now with the velocity, we can calculate the kinetic energy
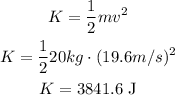
Now let's calculate the potential energy after 2s. Let's first calculate the displacement after that time
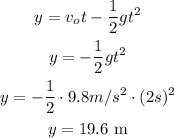
Now let us proceed to calculate the potential energy
