Question

A satellite with a mass of 200 kg fires its engines to increase velocity, therebyincreasing the size of its orbit about Earth. As a result, it moves from acircular orbit of radius 7.5 × 106 m to an orbit of radius 7.7 x 106 m. What isthe approximate change in gravitational force from Earth as a result of thischange in the satellite's orbit? (Recall that Earth has a mass of 5.97 x 1024 kgand G = 6.67 x 10-¹1 N-m²/kg².)A. -59 NB. -112 NC. -73 ND. -32 NSUBMIT

Answer 1

Given:

The mass of the satellite is m = 200 kg

The initial radius of the circular orbit is

`r_i=7.5*10^6\text{ m}`

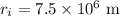
The final radius of the circular orbit is

`r_f=7.7*10^6\text{ m}`

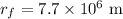
The mass of the earth is

`M=5.97*10^(24)\text{ kg}`

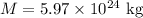
Also, the gravitational constant is

`G=\text{ 6.67}*10^(-11)\text{ N m}^2\text{ /kg}^2`

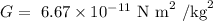
To find the approximate change in gravitational force.

Step-by-step explanation:

In order to calculate the approximate change in the gravitational force, we have to find the difference between the initial and final gravitational forces.

The initial gravitational force can be calculated as

`\begin{gathered} F_i=(GmM)/((r_i)^2) \\ =(6.67*10^(-11)*200*5.97*10^(24))/((7.5*10^6)^2) \\ =\text{ 1415.82 N} \end{gathered}`

The final gravitational force can be calculated as

`\begin{gathered} F_f=(GmM)/((r_f)^2) \\ =(6.67*10^(-11)*200*5.97*10^(24))/((7.7*10^6)^2) \\ =1343.23\text{ N} \end{gathered}`

The approximate change can be calculated as

`\begin{gathered} \Delta F=F_f-F_i \\ =1343.23-1415.82 \\ =-72.6\text{ N} \\ \approx-73\text{ N} \end{gathered}`

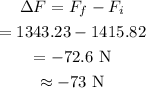
Final Answer: The approximate change in the gravitational force is -73 N