Question

I need help with this practice problem solving It asks, does the series converge or diverge? At the bottom of the pic is question #2, choose one answer from the list provided

Answer 1

Given:

`(1)/(4)+(1)/(3)+(4)/(9)+(16)/(27)+(64)/(81)+.......`

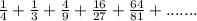
Required:

To find whether the given series is convergent or divergent.

Step-by-step explanation:

The given series is geometric series.

And the common ratio r is,

`\begin{gathered} r=((1)/(3))/((1)/(4)) \\ \\ =(4)/(3) \end{gathered}`

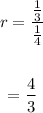
As

`|r|\ge1`

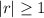
that means the terms do not tend towards 0, as

`n\rightarrow\infty`

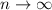
Therefore, the series is divergent.

Final Answer:

The given series is divergent, because the series is geometric and the absolute value of the common ratio is greater than 1.