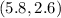Question

Point D is at (1,5) and point G is at (13,-1). If O is on the segment DG, and DO is twice as long as OG, what are the coordinates of O?

Answer 1

You know that:

`\begin{gathered} D\mleft(1,5\mright) \\ G\mleft(13,-1\mright) \end{gathered}`

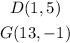
Since the DO is twice as long as OG, you can set up that:

`DO=2OG`

You can find the length of the segment DG by applying the formula to calculate the distance between two points:

`d=\sqrt[]{(x_2-x_1)^2+(y_2-y_1)^2}`

Then, if you set up that:

`\begin{gathered} x_2=13 \\ x_1=1 \\ y_2=-1 \\ y_1=5 \end{gathered}`

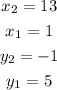
You get:

`DG=\sqrt[]{(13-1_{})^2+(-1-5)^2}=6\sqrt[]{5}`

Now you can set up that:

`\begin{gathered} DG=DO+OG \\ DG=2OG+OG \\ DG=3OG \\ \\ \frac{6\sqrt[]{5}}{3}=OG \\ \\ OG=2\sqrt[]{5} \end{gathered}`

See the diagram below:

Then, using the information provided in the exercise and the values calculated, you can set up the following equation for the x-coordinate of the point O. By definition, the formula to find the coordinates of a point that is located between two points, that has a "r" proportion

`x=((rx_2+x_1))/(1+r)`

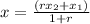
And for "y":

`y=\frac{ry_2_{}+y_1_{}}{1+r}`

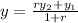
In this case, you know that:

`DO=(2)/(3)DG`

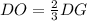
Then:

`r=(2)/(3)`

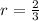
Therefore, substituting this value into each equation to find the coordinates of O, and evaluating, you get (remember to use the endpoints of DG):

`x=\frac{((2)/(3))(13)_{}+1}{1+((2)/(3))}=(29)/(5)=5.8`
`y=\frac{((2)/(3))(-1)+5_{}}{1+(2)/(3)}=(13)/(5)=2.6`

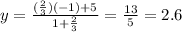
Then, the answer is:

`(5.8,2.6)`