Question

Are these the correct answers? Also, these are from a homework practice

Answer 1

`\begin{gathered} 1)5\pm\sqrt[]{13} \\ 2)D \end{gathered}`

1) We can rewrite that equation to solve it in a clear way:

`\begin{gathered} x^2+12=10x \\ x^2-10x+12=0 \end{gathered}`

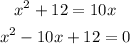
This way we can clearly see the coefficients. Let's solve that quadratic:

`\begin{gathered} x=\frac{-b\pm\sqrt[]{\Delta}}{2a}=\frac{10\pm\sqrt[]{100-48}}{2}= \\ x_1=5+\sqrt[]{13} \\ x_2=5-\sqrt[]{13} \end{gathered}`

2) To find out the number of solutions for this equation, let's calculate the

value of the discriminant:

`\begin{gathered} \Delta=(-5)^2-4(3)(19) \\ \Delta=25-228 \\ \Delta=-203 \end{gathered}`

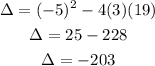
Whenever we have a negative value for the discriminant then we have Complex roots

3) Hence, the answer is:

`1)5\pm\sqrt[]{13}`

2) 2 Complex (Nonreal) Roots