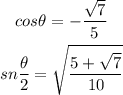Question

Let sin(θ) = (3 times radical 2)/5 and pi/2 < θ < pi.Part A: Determine the exact value of cos 2θ.Part B: Determine the exact value of sin(θ/2).

Answer 1

Final Answer:Given: The trigonometric function

`sin\theta=(3√(2))/(5)\text{ and }(\pi)/(2)<\theta<\pi`

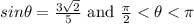
Required: To determine the exact value of

`cos2\theta\text{ and sin\lparen}(\theta)/(2))`

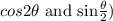
Explanation: Using the trigonometric identity.

`cos2\theta=1-2sin^2\theta`

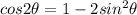
We get,

`\begin{gathered} cos2\theta=1-2*((3√(2))/(5))^2 \\ =1-(36)/(5) \\ =-(31)/(36) \end{gathered}`

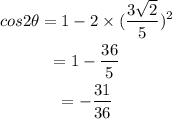
Since theta lies in the second quadrant cos will be negative.

Now,

`\begin{gathered} cos\theta=√(1-sin^2\theta) \\ =\sqrt{1-(18)/(25)} \end{gathered}`

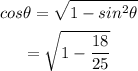
which gives

`cos\theta=-(√(7))/(5)`

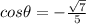
And,

`cos\theta=1-2sin^2(\theta)/(2)`

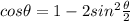
or

`sin(\theta)/(2)=\sqrt{(1-cos\theta)/(2)}`

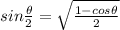
Putting the values we get

`sin(\theta)/(2)=\sqrt{(1+(√(7))/(5))/(2)}`
`sin(\theta)/(2)=\sqrt{(5+√(7))/(10)}`

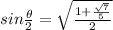
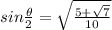
Final Answer:

`\begin{gathered} cos\theta=-(√(7))/(5) \\ s\imaginaryI n(\theta)/(2)=\sqrt{(5+√(7))/(10)} \end{gathered}`