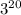Question

If the expression 81^5 was written with a base of 9, which would be the equivalent expression?

A) 9^20
B) 9^25
C) 3^20
D) 3^25

Answer 1

If the expression 81^5 was written with a base of 9.
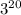`\(3^(20)\)` would be the equivalent expression.

Therefore, correct option is C)
`\(3^(20)\)`

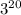
Step-by-step explanation:

To express
`\(81^5\)` with a base of 9, we need to rewrite 81 as
`\(9^2\)`, because
`\(9^2 = 81\)`. So,
`\(81^5\` becomes
`\((9^2)^5\)`, which simplifies to
`\(9^(10)\)`. Therefore, the equivalent expression is
`\(9^(10)\)`, corresponding to option (C)
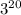`\(3^(20)\)` after further simplification.

Understanding exponent properties, particularly the relationship between bases, allows us to manipulate expressions and find equivalent forms.

Exploring exponent properties, such as rewriting expressions with different bases, is crucial in algebra and higher-level mathematics, providing tools for simplifying and solving complex equations.

Therefore, correct option is C)
`\(3^(20)\)`