Question

Consider that lines u and vare parallel. Which equation models the relationship between the angles? What is the value of x?

Answer 1

Correct option :

`\color{plum}\tt \: \bold{(A) 12x - 4 = 10x + 10 ; x = 7}`

Steps to derive the correct option :

Given :

• Lines u and v are parallel
• Measure of an angle = 12x - 4
• Measure of it's corresponding angle = 10x + 10

Since these angles are corresponding angles and lie on two parallel lines, their value will be equal.

Which means :

`= \tt12x - 4 = 10x + 10`

`= \tt12x - 4 - 10x = 10`

`= \tt12x - 10x = 10 + 4`

`= \tt2x = 14`

`=\tt x = (14)/(2)`

`=\color{plum}\tt x = 7`

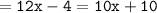
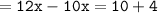
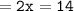
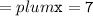
Thus, the value of x = 7.

Let's place 7 in the place of x and see if their value matches :

• 12x - 4

`= \tt12 * 7 - 4`

`= \tt84 - 4`

`= \tt80`

Thus, ∠12x - 4 = 80°

• 10x + 10

`=\tt 7 * 10 + 7`

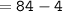
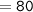
`= \tt70 + 10`

`= \tt80`

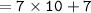
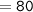
Thus, ∠10x + 10 = 80°

Since the value of these two angles are equal, we can conclude that we have found out the correct measure of each angle.

Therefore, the correct option is - (A) 12x - 4 = 10x + 10; x = 7